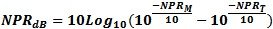By Joseph L. CahakCopyright 2013 Sunshine Design Engineering Services
In today's
world there is a lot of noise and interference. Noise is the cause of many communication, control and instrumentation
problems and inaccuracies. This noise and interference comes from a variety of sources and has a broad spectrum of
dispersion depending on the conditions of the source and the load. Einstein first brought to our attention the effect
the motion of atoms or molecules have on dust particles. This was called Brownian motion. It shows the random motion
of particles due to random light photons activate the electron shells of the molecular groups that then move and bump
the dust particles. This random action and the loss of energy is part of the story of noise and entropy.
Types of Noise
There are many types of noise, Shot noise, Flicker noise, Burst noise, Transit-time
noise Avalanche noise and Thermal noise to name some. See more on these at
Wikipedia Electronic Noise. In addition
to the thermal background noise, the environment is subjected to additional radiation noise from other sources, the
sun, other cosmic objects. Solar radiation pulses impact the earth's magnetic field and influence noise into electrical
systems thru magnetic interactions. Terrestrial sources such as electric devices, motors, generators, switches, surface
contact, sparks or lightning can cause impulse noise. Our cars, the fire on the stove and every sources of heat or
electric power also creates noise radiation in thermal losses. Our primary focus for this article will be Thermal
Noise. Noise is a random distribution of energy with differing energy and time of transition components, this adds
up to a broad frequency distribution of signal energy known as entropy.
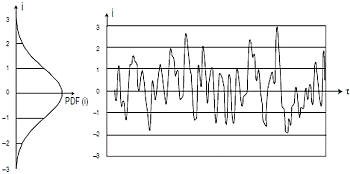
Figure 1 - Random Thermal Noise Spectral Distribution - courtesy Agilent

Figure 2 - Noise Spectral Distribution for Complex Modulation Signals - courtesy Agilent
Spectral Noise
Density
Spectral Noise Density is the noise power per unit bandwidth. Expressed in Watts/Hz or dBm/Hz
it represents the base noise power per unit Hz. Noise power computed for any bandwidth or temperature uses the formula
 to convert between Noise Power and Temperature. See figure3 for Te/Np curve.
to convert between Noise Power and Temperature. See figure3 for Te/Np curve.
Units are typically Kelvin for
temperature and dBm/Hz for logarithmic power/bandwidth or Watts/Hz for linear power/bandwidth. Power for other bandwidths
can be computed from the formula
 .
.
Bw1 in this case being 1 Hz and Bw2 the bandwidth the power total NpdBm
covers.
If we look at the black-body radiation in figure 4, it shows that the photon energy distribution as
a function of wavelength, and inversely the frequency, flattens out at the lower frequencies (longer wavelengths).
So while the output distribution is more Pink Noise as the level is not flat, it is close to flat in the electronic
transmission frequencies. So we assume the Spectral Noise density at RF and Microwave frequencies is relatively flat
at the lower thermal temperature levels. This may not always be assumed. The operator must measure the spectral signal
environment to understand if any interfering signals are present for the noise figure measurement to compute properly.
The shape of the spectral noise density as a function of frequency determines the color of the noise. The main colors
of noise are white, pink, red (Brownian) and grey.
White Noise has a flat distribution of
energy across frequency
 .
.
Pink Noise, also known as flicker noise, has the mathematical representation of
 .
It has a decreasing energy per Hz as frequency increases.
.
It has a decreasing energy per Hz as frequency increases.
Red or Brownian Noise has the mathematical
representation of
 .
It has faster decreasing energy per Hz as frequency increases as pink noise. These first three noise colors are considered
power-law noise as they follow a power law noise energy/frequency distribution White having a 0 exponent, pink a 1
and Brown an exponent of 2.
.
It has faster decreasing energy per Hz as frequency increases as pink noise. These first three noise colors are considered
power-law noise as they follow a power law noise energy/frequency distribution White having a 0 exponent, pink a 1
and Brown an exponent of 2.
Grey Noise is random noise that has been frequency balanced to
psycho-acoustically sounds flat in frequency distribution to our ears. The reader can look up
Colors of Noise on Wikipedia for more information.
There are a number of lesser color variants green, blue, violet, azure, black and more.
Noise Power
To measure noise a reference noise source is used.
There are a number of means to produce noise for reference sources. Among these are Black-body Thermal, Plasma, Electronic
and Digital. They are all , except the digital case, based on the Noise power formula Np=kTeB or
Temperature in Kelvin, Bandwidth in Hertz and Boltzmann's constant. Conversely the noise temperature can be computed
from the noise power.

These Noise power formulas are fundamental to noise sources. While the electronic noise sources may not be
at a real thermal temperature in all cases, they are electrically producing noise at a higher effective noise temperature.

Figure 3 - Noise Power - courtesy Agilent
Boltzmann's Constant
The Boltzmann constant
relates how much energy something has to its temperature and is in units of Joules/K. Currently, Kelvin scale is based
off the triple point of water, which happens when water can exist as a solid, liquid, and gas at 0.01 C or 273.16 K.
The most recent value for the Boltzmann constant is
 =
=
 in SI base units with an uncertainty of Ur=0.71ppm about half of the last best measurement. See
Refining the Kelvin for more information on how they did it or
Boltzmann constant on Wikipedia.
in SI base units with an uncertainty of Ur=0.71ppm about half of the last best measurement. See
Refining the Kelvin for more information on how they did it or
Boltzmann constant on Wikipedia.
Black-Body Thermal Noise Sources
Most everyone today has heard of the Big Bang. This Big
Bang is the concept of the initial point our universe started from. It was an extremely high temperature and infinitely
small, much, much smaller than an atomic nucleus. This expanded to the universe we see today and the Cosmic Microwave
Background radiation is what we see, our rather hear today that is what is left of the energy of the Big Bang. This
energy having expanded and cooled has an average temperature of just under 3.0 Kelvin. Figure 4 shows the change
in radiation wavelength distribution for different temperatures and figure 5 shows the noise density at lower thermal
temperatures. Figure 6 shows the spectral color change with temperature. For the 3 K CMB the spectrum will be
spread to the very low microwave wavelengths and will be very flat in power vs. frequency distribution.
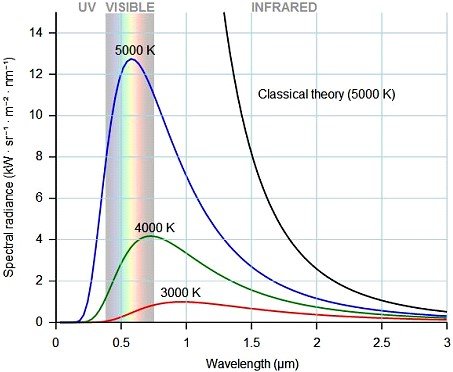
Figure 4 - Blackbody Radiation – courtesy Wikipedia
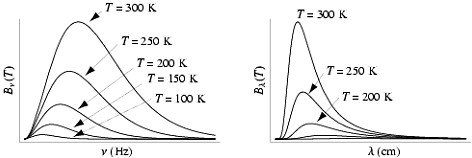
Figure 5 - Wein's Law at Lower Temperatures
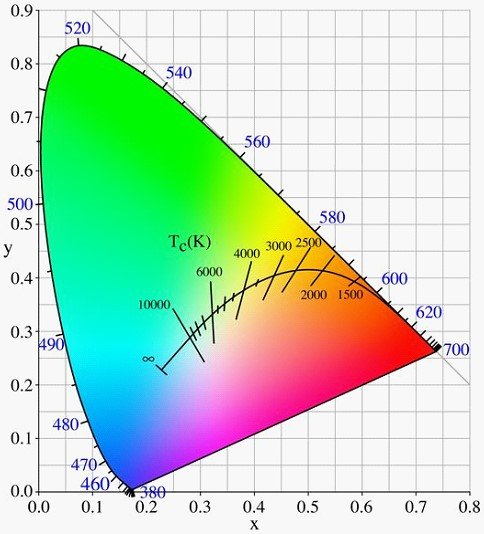
Figure 6 - Color Chart – courtesy Wikipedia
Wein's Law formula can be used to calculate the wavelength peak
of the black body radiation.

For 3 K this works out to 0.000966 M wavelength or about 30 GHz as the peak frequency of thermal
radiation from cosmic background. This puts the cosmic background radiation right in the HF, VHF, UHF thru all the
Microwave frequency range. The Planck Satellite has Microwave receiver bands between 80 and 1000 GHz depending
on the sensors.
As testament to the fact that RF and Microwave frequencies are included in this power curve
of black-body radiation, in some cases a light bulb on a DC circuit with RF coupling can be used as a RF/Microwave
source. See figure 7 for an example circuit. You only need to set the correct current for a certain temperature to
calibrate to specific noise power outputs or ENR (Excess Noise Ratio) compared to cold noise power for the same impedance.
The spectrum of the output power vs. frequency is relatively flat like white noise in the RF and Microwave region.
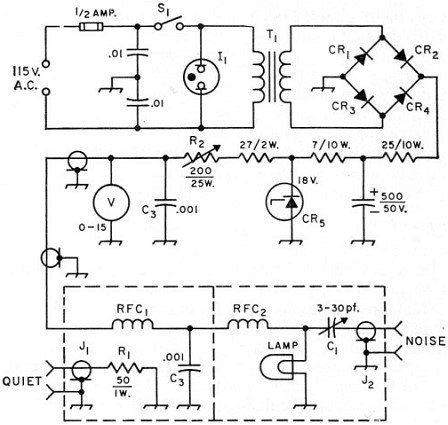
Figure 7 - Lamp Noise Source - see monode reference
Plasma Noise Sources
A plasma source
is similar to the light bulb source, but uses the effective temperature of the plasma instead of the filament. These
ENR's can be very high compared to other sources. An amateur plasma source would be a neon light. Just capacitively
RF couple the noise off the plasma bias circuit for an easy noise source. This circuit would use a very similar circuit
to figure 6, just using higher voltage to drive the plasma lamp.
Electronic Noise Sources
This would include Zeners, avalanche and DSP methods of generating noise output with an electrical bias input.
Electronic noise sources available to amateurs include Zeners which can be used for a noise source for a antenna noise
bridge, impedance bridge, noise source and more. Numerous digital signal (DAC) sources can generate pseudo-white noise
that can be used for test purposes. I will go more into this later. The professional test noise sources more typically
use a form of avalanche diode biased at a specific current level and temperature. Again the noise power is coupled
off the bias circuit with an RF Coupling capacitor or DC coupled as in this case.
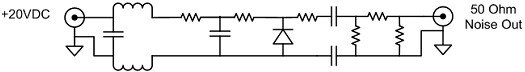
Figure 8 - Schematic of a Typical Diode Noise Source
DSP or Digital Noise Sources
Digitally
generated noise is readily available these days from many Function Generators and can generate complex signals with
added noise for C/N testing and other uses. These can greatly reduce costs for DSP SINAD and other performance test
tools as we will see later.
Noise Calculations
Given that any device that carries electricity generates heat, it also creates radiated noise power. In amplifiers
we call this amplifier generated noise its noise figure. This gives us a measure of the signal to noise degradation
of signals received and desired to be measured.
ENB – Effective Noise Bandwidth
ENB
or Effective Noise Bandwidth is the effective power integration bandwidth or envelope of the noise power integration
for total power across the spectrum. This is the ratio to correct for the effective Resolution Bandwidth of the Spectrum
Analyzer or Power Measurement device with frequency selectivity to eliminate spurious signals and give an equivalent
power integration bandwidth. Agilent typically uses 1.2 as the ENB integration scaling factor applied to the Resolution
Bandwidth or RBW. When the Spectrum Analyzer operator selects Noise Marker the bandwidth correction is made for you.
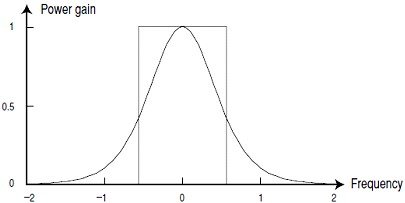
Figure 9 - ENB Effective Noise Bandwidth - courtesy Agilent
Signal to Noise Ratio
The
S/N or Signal to Noise ratio is a dimensionless power ratio. It is the signal amplitude in watts compared to the Noise
power level in watts at signal off conditions. This gives some measure of signal quality or at least signal detection
capability. Every network that transports energy, in this case the RF/Microwave region has a loss or noise increase
with gain and this equates to a degradation of the S/N ratio. This measure of signal to noise degradation is called
the noise factor. This is in linear numeric scale and units relate to power ratios or signal to noise.
 .
The
.
The
 is the signal to noise ratio of the system calibration. The
is the signal to noise ratio of the system calibration. The
 is
the signal to noise ratio of the system and DUT total response. This is more typically used in the Log format Noise
Figure in NFdB which can be computed from the formula
is
the signal to noise ratio of the system and DUT total response. This is more typically used in the Log format Noise
Figure in NFdB which can be computed from the formula
 .
The inverse of this can be computed from
.
The inverse of this can be computed from
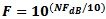

Figure 10 - Signal to Noise Degradation courtesy Agilent Technologies

Figure 11 - Signal to Noise Degradation vs. Temperature Effective in Kelvin - courtesy Agilent
Minimum
Detectable Signal
Minimum Detectable Signal is determined by the receiver Noise Figure.
Np=kTB for T=290 K = 4*10-21 watts or -174 dBm/Hz. This is the minimum energy
in noise for a broad white spectrum distribution in the RF and Microwave region. This can also be converted from power
to noise volts in Vrms units or Arms noise current Vrms =
 or Irms =
or Irms =

We now we have a basis for
 or
or
 when
the temperature of the system under test is not at Te=290K.For a receiver with a S/N performance level the MDS formula
adds the S/N factor
when
the temperature of the system under test is not at Te=290K.For a receiver with a S/N performance level the MDS formula
adds the S/N factor

Tangential Sensitivity or TSS
A related signal detection parameter Tangential Sensitivity
or TSS can be computed from
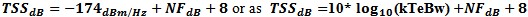
Noise Temperature
Noise factor is related to noise temperature by
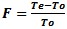 or
or
 or Te = To(F-1).
or Te = To(F-1).
To=cold temperature and Te is effective temperature both in Kelvin.
 for when user is using noise figure in log units.
for when user is using noise figure in log units.
From this we can see that to figure to a positive F, Te must
exceed To. Cold Noise To depends on the desired signal to be measured. Terrestrial thermal noise limits us to greater
than approximately 290K or ambient temperature of the environment. For deep space signals from gaseous clouds or other
cold space targets, one would need close to 0K cooled receivers to detect the signal powers from space without being
swamped by receiver noise, thus they use liquid helium coolers on these sensitive space microwave receivers. They
use a 0.1K liquid He3 for the Plank satellite to measure the 3K Microwave background radiation.
ENR or Excess Noise Ratio
A noise source is rated by its port match and the ENR or excess
noise ratio of the source and the ENR table vs. frequency calibration table. This ENR is typically expressed in dB
units and is formulated from the Te and To values for the source.
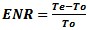 or
or 
 or
or  with
To=290K
with
To=290K
Y-Factor
Y-Factor method uses the spectral analysis method to measure the spectral
density as a function of frequency and get the noise power in a specified noise bandwidth and comparing it to a cold
noise power measurement at the same bandwidth condition. This Y factor or ratio is ratio of the noise on to noise
off power ratio or
 off with Np on must be greater than Np off to compute a noise figure. Then the Y ratio can be used to compute the
Noise Figure in dB. Be sure to use sample detector mode when making these measurements. Video Averaging will reduce
the specular noise by averaging across frequency to smooth to the video average.
off with Np on must be greater than Np off to compute a noise figure. Then the Y ratio can be used to compute the
Noise Figure in dB. Be sure to use sample detector mode when making these measurements. Video Averaging will reduce
the specular noise by averaging across frequency to smooth to the video average.
 or
or 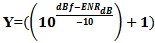
dY/dENR
Another variant of the Y factor method that does not require a calibrate noise
source is the dY/dENR method. The method uses the Y and ENR measurements of 2 noise levels with and without the DUT.
The formula uses the 1st derivative of the
 from
which we derive
from
which we derive
 .
.
Note that is requires 3 or 4 measurements to get the dY and dENR and each of these measurements has error,
especially if not using a good spectrum analyzer with a low noise input and level of detection.
Noise
Measure
Noise Measure is a measure of the noise quality of the part when noise factor and gain are
both considered to an infinite extension of the cascade equation, e.g. it is a measure of the system performance limit.
 in
linear units of F=Noise Factor and G=Gain in linear units.
in
linear units of F=Noise Factor and G=Gain in linear units.
Receiver Noise Power Input
When making measurements or using a receiver for signal detection, the user must pay attention to the total power
input to the receiver or detector/sensor to prevent overload of the receiver/sensor. Receiver gain includes antenna
gain, preamp gain and total down-converted stage gain. Small signal level of broadband modulated signals can easily
swamp receiver front ends.
Np=kTeBG in Watts/Hz which can be converted to spectral noise
density dBm/Hz. The added G factor for gain is to account for the total system noise power after receiver system gain.
For wide bandwidth modulations like UWB and QAM >128 the channel power for low signal levels can be quite large
and overload Spectrum Analyzer front ends.
DANL or Displayed Average Noise Level
This
gives the relationship between the DANL of the spectrum analyzer and the analyzer noise figure of its receiver. The
1.2 is a bandwidth ENB or effective noise bandwidth factor to account for effective power integration bandwidth.

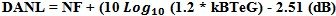
Signal to Noise Level Power Average Error
When measuring CW signals close to the noise
floor, as Power (mW or W) and you measure the signal and noise power and remove the signal and measure just the noise,
you can figure what the actual signal power is without the added noise power. This is true even if the power is displayed
as dBm.


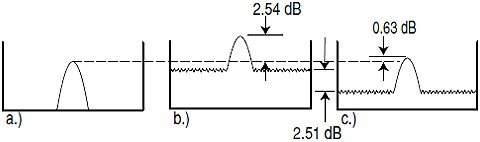
Figure 12 - Signal to Noise Measurement Error - courtesy Agilent
Signal to Noise Level Log Power Average
Error
When measuring CW Signals that are close to the noise floor and using log averaging the statistics
are different and noise has less effect on the measurement error. The formula to figure the Signal error from the
Signal and Noise total and the S/N delta in dB will give the Corrected Signal level. Agilent is using this formula
and the individual noise characterization of each PSA and PXA to compute actual signal power down into the noise floor.
Seemingly pulling these signals out of the Spectrum Analyzer DANL thermal noise floor is part of what Agilent is calling
Noise Floor Extension.
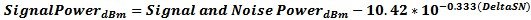
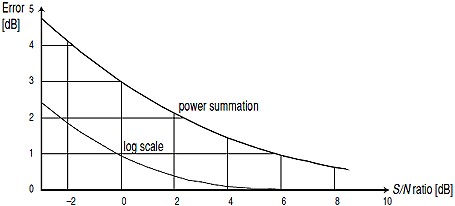
Figure 13 - Signal to Noise Log Power Average Error - courtesy Agilent
Noise Floor Extension – Agilent
PSA/PXA
Agilent Technologies PXA Spectrum Analyzers offer a new feature called Noise Floor Extension
or NFE. This technique requires a full spectral noise density characterization of the instrument. Agilent uses the
Power Average or Log Average Noise Correction techniques previous to pull the noise floor down and the signal out
of the noise.
Quantization Noise Error
When measuring analog signals with Analog to
Digital converters or generating analog signals with a DAC, there is a level of noise degradation based on the level
of resolution of the ADC/DAC. The amount of the signal left after the digital “bit” voltage.
The formulas for
Sine or Arbitrary Wave forms are:
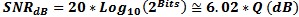 for
Arbitrary Waveforms
for
Arbitrary Waveforms
 for
Sine Waveforms
for
Sine Waveforms

Figure 14- Quantization Noise Error
Cascaded Noise Figure
To compute the noise figure
of cascaded network sections, the following formula is used. In noise factor and linear gain units the formula is:

For the 2 stages case:
 or
or  or
or 
Use
 to convert to dBF. This can be used to compute the noise figure of a DUT embedded inside a test fixture. Keep in mind
T can be substituted in for F using Te=To(F-1) in the equation for cascaded noise calculations. We will see these
formulas again with input and output loss correction of DUT measurements. We will also see this in the cold power
noise measurement technique.
to convert to dBF. This can be used to compute the noise figure of a DUT embedded inside a test fixture. Keep in mind
T can be substituted in for F using Te=To(F-1) in the equation for cascaded noise calculations. We will see these
formulas again with input and output loss correction of DUT measurements. We will also see this in the cold power
noise measurement technique.
In addition the equivalent Temperature cascade formulas are


Figure 15 - Cascade Noise Figure - courtesy Agilent
Temp Corrected Noise Figure of Passive Loss
With a Loss in linear units and a Ta ambient temperature the Te effective temperature of the loss is
 from
which we compute the noise factor
from
which we compute the noise factor
 .
.
Use NFloss = 10log10(F) to get the loss at temperature in dB. Tl
= loss temperature in Kelvin.
Temp Corrected ENR
The temperature corrected ENR
of a calibrated Noise source at a temperature other than 290 K can be computed to correct for the actual temperature
offset error factor.

ENR= Noise Source ENR in dB from calibration table
Ts=Noise Source actual temperature
To=290 K cal
temp
Temp Corrected Y-Factor Noise Figure Measurement
The formula for temperature corrected
Y-factor noise figure measurements is

where

and

Mismatch Loss for Noise Source
 . The + and – represent the max and minimum mismatch for the measurement mismatch loss of power measured. ρg and
ρl are the generator and load reflection coefficient. This would be an input loss direct error factor
on the measurement that is F1 in the cascade noise equation along with the input network loss added for the total
loss.
. The + and – represent the max and minimum mismatch for the measurement mismatch loss of power measured. ρg and
ρl are the generator and load reflection coefficient. This would be an input loss direct error factor
on the measurement that is F1 in the cascade noise equation along with the input network loss added for the total
loss.
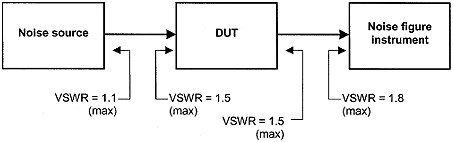
Figure 16 - Noise Figure Measurement Mismatch Effects - courtesy Agilent
Input Loss Correction
The input loss can be applied as a first order correction along with the mismatch loss for the ENR correction
accounting for the input loss factor decrease in the ENR of the noise source. This is a first order correction and
not as accurate s the Available Gain/Loss method described shortly. The operator must also remember to add system
gain in the measurement output path into the NFM to overcome any input loss. If this is not accounted for, the noise
figure meter may fail to calibrate.
A first order correction can be as simple as subtracting the Effective
Loss NF dB from the ENR in dB.
This does not account for match or other corrections. Make sure Input Network
LossdB is corrected for actual F at temperature K.

Figure 17 - Noise Measurement with Input Loss

To correct the DUT temperature for the input loss of the DUT fixture system use the equation
 T1=DUT, Lin = Loss, Tl = Loss effective temperature in K.
T1=DUT, Lin = Loss, Tl = Loss effective temperature in K.
Output Loss Correction
The Cascaded Noise equation can be used to compute the output stage on the total system noise level into the receiver
and account for this stage of loss/gain. If the device under test has a >10 of gain, then the output stage loss
only impacts the gain more than the noise figure. This changes if the device under test has low gain or is lossy.
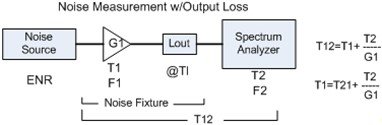
Figure 18 - Noise Measurement with Output Loss
To correct the DUT temperature for the output loss of the DUT
fixture system use the formulas:
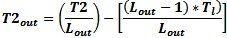
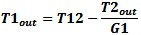
Combined Input and Output Loss Correction
Combining the previous equations for input
and output loss to be applied to noise figure measurements on a Noise Figure Meter when applying the input and output
losses (or matching losses) to the corrected noise path measurement. The NFM would be calibrated without these losses
and these input and output losses added to the DUT would be applied to the measured results to correct for the losses.
This de-embeds the DUT NF from the test fixture which comprises the input loss, DUT and output loss being measured
by the calibrated NFM.
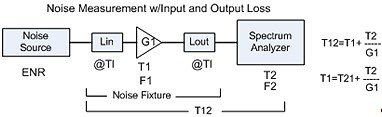
Figure 19 - Noise Measurement with Input and Output Loss
 n
n
T1In/Out is the corrected DUT temperature for the input and output loss
of the DUT fixture system.
Input Network Available Gain/Loss
The user can use a first
order correction of the loss in dB and the mismatch loss of the input network. A more accurate method would be to
calculate the available gain/loss from the input network s-parameters. Available gain/loss is the ratio of the power
available from the 2-port network to the power available from the source. This gain is useful to calculate the network
gain (or loss) of an input network to a device being tested for noise figure. This loss is the noise figure in dBF
of the input network for the cascaded gain equation when making a device noise figure measurement.
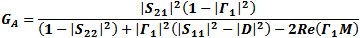
with
D = S11S22-S21S12
M = S11-DS'22
N = S22-DS'11
Noise Power Ratio
Noise Power ratio
gives a measure of the Infinite Intermodulation Performance of networks to digital wideband signals. It is a measure
of the degradation of the measured vs. the reference test signal.

Noise Measurements
Noise Measurements of
Linear Devices or Non-Frequency translating
To measure a typical electronic sub-assembly as an amplifier,
a passive network or any other active device assemblies that are not frequency translating, the operator has several
methods available to make a noise measurement. They can either use a Noise Figure Meter or a Spectrum Analyzer or
a power meter with some form of filtering and amplification for the intended measurement frequency and power bandwidth
and low power signal levels of noise.
Noise Figure Meters
Noise Figure Analyzers are
basically high quality power meters with front end frequency filtering. The older Agilent 8970 series noise figure
meters had a fixed 4 MHz bandwidth that it measured noise power over. If there were any interfering signals,
noise figure accuracy could be greatly impacted. Noise Figure could also be impacted by narrow frequency response
of the DUT affecting the power integration bandwidth. The newer Noise Figure Analyzers from Agilent have a 100 kHz
to 4 MHz selectable measurement bandwidth. This does help some, but not completely eliminate issues with noise
figure measurements with interfering signals and narrow bandwidth. The Noise Figure meter works best with the noise
signal bandwidth being flat through the NFA bandwidth range. If sub-assembly filters or other bandwidth restricting
components are within the DUT it will compromise the Noise Figure Measurement. In this case the noise figure power
integration bandwidth should be less than or equal to the minimum device under test bandwidth. This will eliminate
the unequal noise integration bandwidth issues of cascaded sections. The drawback is the need for amplification to
overcome lower power readings with narrower bandwidth, which will add more uncertainty to the measurement.

Figure 20 - Typical Noise Measurement System - courtesy Agilent
An issue for noise figure meters is they do
not work well with much loss between the noise source and the device under test. In practice I found about 1-2 dB
loss was enough to give the 8970B NFM difficulty calibrating. One method to overcome this is to put a preamp in front
of the noise figure meter to boost the noise power level to compensate for the input loss. This will result in loss
of test system dynamic measurement range and possible input power saturation or overload. One needs to either measure
(best) or compute the noise power into the receiver before connecting. We do not want to destroy sensitive power measurement
equipment or overload it and get bad measurements.
The noise measurement could be performed with a filter pre-amp
and a power sensor, but is limited by the filter frequency and bandwidth and the pre-amp NF and Gain and the sensitivity
of the power sensor all being fixed values. This should be an RMS or True power sensor. So this test topography could
be made using other instruments besides the Noise figure meter to do the same thing. I've use everything from a power
meter, Spectrum Analyzer, Vector Network Analyzer to a Scalar Analyzer for various customer/clients.
Noise
Figure test issues are typically boiled down to dynamic range (hi or low end gain) or spurious signals. If having
trouble measuring noise figure with a noise figure meter, first diagnostic I perform is a spectrum check of the device
output into the NFM and look at the noise on and noise off levels to be sure they are about what I expect to see as
well.

Figure 21 - Spectrum Analysis of Noise Receiver Input - courtesy Agilent
If measuring devices of characteristic
impedance other than 50 Ohms some means of matching the 50Ohm Noise Measurement System to the DUT under test. A Unbalanced
to Unbalanced (UnUn or Coaxial) 50 ohm to 75 ohm could be used for instance, or another choice would be a 50-75 ohm
minimum loss matching pad. This will add about 6 dB of loss to the path. These matching losses must be accounted
for in the cascade noise math to compute the correct noise figure of the DUT, again a preamp into the receiver may
be necessary to have the correct noise power levels for accurate measurements.

Figure 22 - Noise Figure with Matching to DUT - courtesy Agilent
Noise Measurements by Y-Factor Spectral
Analysis
The issues mentioned above using a Noise Figure Meter, such as, spurious signal or detector
dynamic power range are significantly overcome when using a spectrum analyzer. Where the older spectrum analyzers
had slow sweep response and high input noise figures, today's analyzers are much faster, more accurate and have far
better front ends and lower noise figures when Preamps are added to the front ends. Using a spectrum analyzer the
test frequency can be easily moved to quieter areas to make the noise measurement. Many modern spectrum analyzers
have the Y-Factor capability built in and have a drive for the noise source and the firmware to make the calculation.
We begin the Y factor measurement by looking at the spectrum analyzer and its characteristics. First set the spectrum
analyzer to sample detector at the lowest Resolution Bandwidth that gives reasonable sweep time. Place the Spectrum
Analyzer in Video Averaging mode and get the noise floor average across frequency or DANL in dB. This is related to
the Spectrum Analyzer's noise figure and is a measure of the sensitivity of the analyzer.
Knowing the Spectrum
Analyzer DANL and looking at the Noise Source with Noise ON, if we can see the jump in noise floor of the spectrum
analyzer, we know we will be able to measure the Noise On and Noise Off with the DUT in the measurement system provided
the DUT has a reasonable gain (>10 dB). We only need the DUT Noise On and Off measurement and the ENR of the
noise source to compute the Noise Figure.
We know the ENR of the source (Calibration Noise On/Noise Off), so
don't need to measure it. But if the Noise On measurement of the Noise Source is more than 10 dB above the DANL
of the Spectrum Analyzer, you can de-embed a reasonable accuracy on the Noise Source ENR.
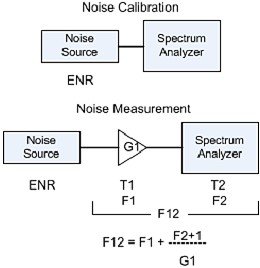
Figure 23 - Spectrum Analyzer Noise Measurement
When measuring a frequency linear device such as an amp, filter,
etc. the ENR used is the interpolated ENR value for the RF frequency of interest ratioed between the ENR table values
above and below frequency measured. They are usually calibrated every GHz maybe 10 MHz and 100 MHz at the
low frequency end.
When measuring frequency translational devices such as Mixers, Receivers, Multipliers the
ENR of the RF input is used and the measurement is made at the IF Freq or Vice-versa if the frequency conversion goes
the other way and there may be sidebands involved or both sidebands. These need to be considered to make the measurement
properly depending on the frequency conversion topology of the DUT.
Noise Measurements of Frequency
Translating (Mixer) Measurements
The Noise Figure Analyzer also makes complex frequency translating
and single sideband measurements much easier. The analyzer takes care of the ENR factors at frequency and also the
LO frequency for the test. When measuring the Noise Figure of a frequency translating device such as a mixer, converter,
doubler, tripler or other means of translating a signal from one frequency to another, a similar technique is used
in that you know the noise power of the source hot and cold at the RF frequency being input or received. Then from
the output Noise On and Noise Off measurement thru the DUT the converted signal noise degradation can be measured.
Even though the measurement is made at a different frequency the power rations are the same and the ENR used is at
the frequency of input to the device under test.
Noise Figure Meters can be used to semi-automate these measurements
by programming in the type of conversion Up-conversion or down-conversion. If the LO being used is above or below
the RF frequencies or the IF being used as the input RF signal. This will determine if the RF, IF or LO are CW or
swept and it the signal being converted to will sweep up or down in frequency. This is all important information for
the proper path loss corrections for the signals input and output for the corrected Noise Figure of the measurement.
In addition, one must know if you are measuring a single or double sideband device. If you are measuring an SSB device
the actual noise figure will be 3dB higher, as you are only measuring half the input signal power.
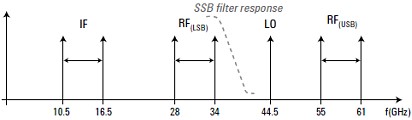
Figure 24 - Mixer Frequency Plan - Fixed LO - courtesy Agilent

Figure 25 – Mixer Noise DSB/SSB Measurements - courtesy Agilent
When using the NFM, filters may be necessary
to eliminate spurious signals. Spectrum Analysis Y-factor is ideally suited for noise figure measurement on frequency
translating devices as spurious signal avoidance is much easier. We re-iterate that when measuring frequency translational
devices such as Mixers, Receivers, Multipliers the ENR of the RF input is used and the measurement is made at the
IF Freq or Vice-versa if the frequency conversion goes the other way and there may be sidebands involved or both sidebands.
These need to be considered to make the measurement properly depending on the frequency conversion topology of the
DUT.
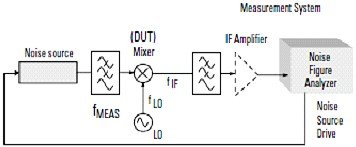
Figure 26 - Mixer Noise Figure Test - courtesy Agilent
Cold Power Noise Measurement
This method uses the basic cascaded noise formula to derive the noise power from an input termination thru the
DUT and into the noise measurement device. By measuring this output power and knowing the input thermal power by the
kTeBw formula for the watts of the defined bandwidth, we can then derive the F for the DUT under test. The high speed
RFIC testers like this method, as it takes a lot less time; even if has bandwidth, match, gain/loss accuracy of switched
paths and contacts repeatability and match etc. It is still very fast. You only measure one cold power sweep, not
one hot and one cold as with the Y-Factor or NFM methods although the accuracy is much worse.
Active
Device Noise Characterization for Design
Part of designing active device gain blocks for low noise
front end amplifiers is to understand the noise and gain characteristics over a range of input matches. By having
a test setup with integrated Vector Network Analyzer for S-Parameters and a Noise Source with input tuning stubs to
match the input of hthe device the Fmin and Rs and constant noise circles and gain circles and center can be determined
to aid designs using the device for better performance. By varying the match and measuring the DUT under the input
match conditions and de-embedding the pre-calibrated tuner network response to get the raw device performance parameters
which can be modeled by the formula. In figure 27 the pink circles of the paraboloid show a different frequency than
the red circles.


Figure 27 - Noise Match Performance Paraboloid – courtesy BSW Inc.
The center of the paraboloid of noise performance
on the smith chart load display is calculated with the formula:

The circles of constant noise performance are calculated with the formulas:

The Noise Factor at each of the circle boundaries:


Figure 28 - Noise Circles on the Smith Chart – courtesy BSW Inc.
Other
Noise Test Methods
Noise Source as Infinite Bandwidth Signal Source
A noise source with an amplifier to increase the noise level can be used as effectively a wideband signal source.
Take the wideband noise power from a noise source and pass it thru a filter, I can integrate the spectral response
by averaging the output on a Spectrum Analyzer and get the filter response envelope within a short time with increasing
accuracy as the averages build in count.
Noise Bridge
The noise bridge is a measurement
instrument using a broadband RF noise source such as Zeners, or resisters, neon lamps or other broadband noise sources.
Then boost the power level with an amplifier and inject into a balanced bridge. The bridge resistance and reactance
tuning for nulling allows the operator to figure a system or antenna characteristic impedance so the antenna can be
balanced for best power transfer. The bridge resistor of 0-250 Ohms and 0-140 pF is balanced against a 70 pF
capacitor and 10 Ohm resistor and the unknown load of the antenna or other network. The 250 Ohms is to balance
against network impedance of typically 20-200 Ohms range and the 140 pF variable tuning capacitor balances
against 70pF in the other arm to give a +/-70 pF reactance tuning range. This gives most communication folks
a good practical and cheap tool to get matching information on networks and their antennas.
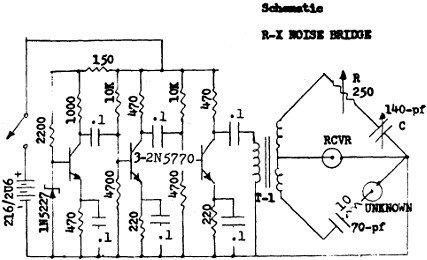
Figure 29 – Palomar Noise Bridge Schematic – courtesy Palomar Technologies
Noise and Antenna Gain
While researching noise measurements I found this info on antenna measurements of interest. The noise spectral
density measured at the antenna output compared to the ambient thermal noise level (kTB) gives a measure antenna gain
factor. Noise being infinite bandwidth this gain factor covers a broad frequency range, so it gives an easy and simple
means to measure the wideband antenna gain performance.
Noise for C/N or Eb/No Transceiver Performance
Measurements
Using an RF Noise source and providing power amplification to produce known level of
noise to couple with signal sources of known level gives the capability to test Transceivers for the Carrier to Noise
level performance testing. There are variants of this that can test the Eb/No (Energy per Bit to Noise Level) when
the data bit rate information is added to the calculation. Eb/No is related to C/N by the equation
 with
ENB=Rcvr Noise BW, fb=bit rate
with
ENB=Rcvr Noise BW, fb=bit rate
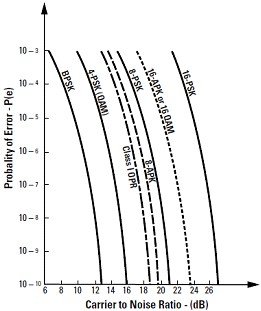
Figure 30 - Carrier to Noise to BER Curves - courtesy Agilent
Noise for Jamming
The
Noise source taken to an extreme with huge power amplifiers can be used to flood an area with high level of false
ambient RF noise and jam all signals in that area with such high Noise levels that most all receiver systems will
fail to operate properly. It is my understanding the services use 750 kW TWT's to flood the engagement areas
with noise to jam. This power of 88 dBF of Noise power is equal to ~1.83*1011 K, so this is a
very hot source indeed.
Signal to Noise (S/N) Measurement
This is a measure of the
signal level over the base average noise floor of the receiver system. The average peak of the signal is compared
to the average noise floor level and the difference is expressed in dB relative difference.
Noise for in-circuit
testing and calibration
Noise can be used as in an in-circuit Built In Self Test or BIT or BIST. This can give
several performance parameters such as S/N, gain an sometimes more for the device the noise injector is built into
for this purpose.
SINAD
SINAD is a means to measure signal to noise and Distortion
quality with a simple instrument. The power of the noise and distortion with the Signal and without to determine the
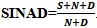 .
In practice the power is detected for the input signal for the S+N+D. For the N+D the signal is filtered with a tight
signal frequency filter and the Noise and Distortion components are left to use to divide the total power by for the
resulting SINAD. When measuring a receiver audio output the bandwidth of the SINAD measuring instrument can give varying
results depending on how wide the noise floor extends in frequency. If the audio output is integrated for SINAD over
a 3 kHz bandwidth vs. over a say 20 kHz bandwidth the SINAD will be much lower for the wideband noise case
verse the narrow band audio noise case. Most receiver audio channels are not very wide in bandwidth for signal
transmission efficiency. So, if having trouble with a receiver, measuring SINAD, check the measurement bandwidth.
In the case shown in figure 31 and 32, the impact of the wideband audio noise on a SINAD measurement is demonstrated.
In this case SINAD went from 16.4 dB in the 20 kHz noise case to 18.4 dB in the 3 kHz noise bandwidth
case. In both cases the S/N ratio was approximately equal. So the difference was just from the added noise bandwidth.
.
In practice the power is detected for the input signal for the S+N+D. For the N+D the signal is filtered with a tight
signal frequency filter and the Noise and Distortion components are left to use to divide the total power by for the
resulting SINAD. When measuring a receiver audio output the bandwidth of the SINAD measuring instrument can give varying
results depending on how wide the noise floor extends in frequency. If the audio output is integrated for SINAD over
a 3 kHz bandwidth vs. over a say 20 kHz bandwidth the SINAD will be much lower for the wideband noise case
verse the narrow band audio noise case. Most receiver audio channels are not very wide in bandwidth for signal
transmission efficiency. So, if having trouble with a receiver, measuring SINAD, check the measurement bandwidth.
In the case shown in figure 31 and 32, the impact of the wideband audio noise on a SINAD measurement is demonstrated.
In this case SINAD went from 16.4 dB in the 20 kHz noise case to 18.4 dB in the 3 kHz noise bandwidth
case. In both cases the S/N ratio was approximately equal. So the difference was just from the added noise bandwidth.
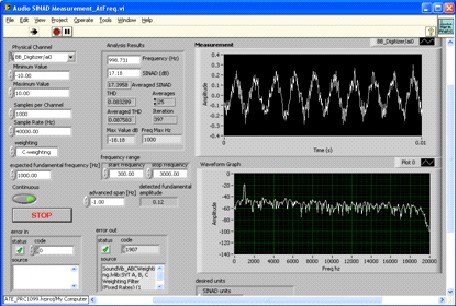
Figure 31 - 20 kHz Audio Noise SINAD

Figure 32 - 3 kHz Audio Noise SINAD
Noise Power Ratio
This measurement can be
useful in specialized cases for measuring and determining spectral regrowth of broadband digital systems (amps, receiver,
transmitters, etc.). The spectral emissions masks of most communication standards have spectral masks that must be
met to be approved for use. To do this, amplifiers and other devices have to control spectral regrowth from intermodulation
products.
A Noise Power Ratio Analyzer creates a noise source, with a deep notch band reject filter to cut
a gap of some bandwidth in the broadband noise sources. When this reference test signal is sent thru the device under
test, the intermodulation products from this infinite source created Infinite Intermodulation Products IIP's that
fill the noise gap. The new level with the DUT performance is compared to the old notch level gives a measure of the
IIP performance and an indication of the devices contribution to spectral regrowth.
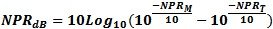
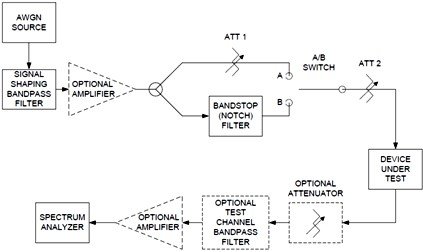
Figure 33 - Noise Power Ration Test System

Figure 34 - Noise Power Ratio Signals
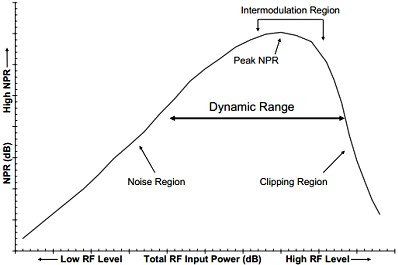
Figure 35 - Noise Power Ratio Performance Curve of Amp - courtesy Agilent
Conclusion
We have reviewed Noise and Noise Measurements and its many uses. We showed many of the Noise formulas and uses
for Noise as well as important uses for noise. We will see new receiver technology to glean weak signals even further
out of the noise background to extend our communication reach even further than is now possible. Voyager1 has
now passed the boundary of the Heliosphere. Our technology to receive these faint signals improves year after year.
We are still digging Voyager's signal out of the noise with Silicon on Sapphire semiconductors and Parametric Amplifiers
that work on near magic to get these infinitesimal signals out of the ether. The technology to detect these signals
did not exist when these spacecraft were first sent up to space.
The measurement of the Cosmic Microwave Background
or CMB may open new knowledge about our universe on the largest scale. Who could have known the reach of noise on
our knowledge? What we started out studying to understand may end up telling us something about our place in the universe,
the fate of that universe. The patterns of noise show us the acoustic signature at the point of rapid inflation at
the birth of the big bang, which created the imprint for future matter (galaxy) distribution of the universe. Scientists
have refined the age of the universe, how fast it is expanding and cosmic constants. The analysis of the size scale
of the noise temperature fluctuations in the CMB tells us about the makeup of the universe and the ratio of Dark energy,
dark matter and ordinary matter. The signatures on noise temperature of the universe are also being looked at for
patterns representing the possibility of multiple universes and partners to ours bumping into our universe.
Clearly noise has much to teach us about many things and is of great use, in addition to being the bane of communications.
Sunshine Design Engineering Services
23517 Carmena Rd
Ramona, CA 92065
760-685-1126
Featuring: Test Automation
Services, RF Calculator and RF Math/Noise and S-Parameter Library (DLL & LLB)
LinkedIn: Joe Cahak
SunshineDesign@cox.net
References
- The "Monode" Noise Generator Hot-Resistor Noise-Figure Measurement By Ronald E. Guentzler, W8BBB
- A Calibrated Noise Source for Amateur Radio by William Sabin QST May 1994
- The ARRL Radio Handbook for Radio Communications 1999 and 2013 editions, ARRL, ISBN:978-0-87259-405-0
- The ARRL UHF/Microwave Experimenters Manual, ARRL, ISBN: 0-87259-312-6
- High-Frequency Circuit Design and Measurements, Peter C. L. Yip Chapman and Hall ISBN: 0-442-31185-0
- Electronics Equations Handbook, Stephen J. Erst, TAB. ISBN:0-8306-3241-7
- Microwave and RF Design of Wireless Systems, David M. Pozar, John Wiley & Sons, ISBN:0-471-32282-2
- Noise Reduction Techniques in Electronic Systems, Henry W. Ott, John Wiley & Sons, ISBN: 0-471-65726-3
- Communication Formulas & Algorithms, C. Britton Rorabaugh, McGraw-Hill, ISBN:0-07-053644-9
- Spectrum and Network Measurements, Robert A. White, Prentice-Hall, ISBN: 0-13-030800-5
- Fundamentals of Spectrum Analysis, Christopher Rauscher, Rohde & Schwarz, ISBN: 978-3-939837-01-5
- Agilent E2507B/E2508A Noise Power Ratio (NPR) Measurements Using the Agilent E2507B/E2508A Multiformat Communications
Signal Simulator Product Note-5965-8533E
- Agilent AppNote:150-4 5952-1147E Spectrum Analysis…Noise Figure Measurements
- Agilent AppNote:57-1 5952-8255E Fundamentals of RF and Microwave Noise Figure Measurements
- Agilent AppNote: 57-2 5952-3706E Measurement Accuracy – The Y-Factor Method
- Agilent AppNote: 57-3 5980-0288E 10 Hints for Making Successful Noise Figure Measurements
- Agilent AppNote : 1303 5966-4008E Spectrum Analyzer Measurements and Noise
- Agilent AppNote : 1484 5989-0270EN Non-Zero Noise Figure After Calibration
- Agilent AppNote : 1439 5988-8571EN Measuring Noise Figure with a Spectrum Analyzer
- Agilent Product Note : 85719A-1 5091-4801E Maximizing Accuracy in Noise Figure Measurements
- Agilent AppNote : 5990-5340EN Using Noise Floor Extension in the PXA Signal Analyzer
- Rohde & Schwarz, Application Note: The Y Factor Technique for Noise Figure Measurements
- Calculate the Uncertainty of NF Measurements, Duncan Boyd, Microwaves & RF October 1999
- Microwave Engineering, David Pozar, Wiley
- Palomar R-X Noise Bridge Operators Manual
- Receiver Noise Figure, Prof. C. Patrick Yue, ECE, UCSB
- Microwave Engineering, David M. Pozar, Wiley, ISBN: 9971-51-263-7
- The Colorful World of Noise, Tom Lecklider, Evaluation Engineering, May 2008
- An Introduction to Noise Figure, Jonathan Bird, RF Design, March 1993
- Noise of Cascaded Two Port Deices of Uneqaul Bandwidth, George J. Bertsche, MSN&CT, May 1988
- Bandwidth Effects in Noise Figure Measurements, William E. Pastori, MSN&CT, April 1988
- Noise Measurement and Generation, Paul Wade, N1BWT, ARRL
- Measure Noise without a Calibrated Source, Roy Monzello, Microwaves & RF, April 2013
- Make Accurate Sub-1dB Noise Figure Measurements Part 1 Noise Concepts., High Frequency Electronics
- Noise Basics www.NoiseCom.com
- www.Noisewave.com
Sunshine Design Engineering Services
is located in the sunny San Vicente Valley near San Diego, CA, gateway to the mountains
and skies. Are you looking for new things to design, program or create and need
assistance? I offer design services with specialties in electronic hardware, CAD
and software engineering, and 25 years of experience with Test Engineering services
in RF/microwave, transceiver and semiconductor parametric test, test application
program development, automation programs, database programming, graphics and analysis,
and mathematical algorithms.
See also:
- RF Connectors and Cables
- Searching for the Q
- Hybrid Heaven
- Noise and Noise Measurements
- Solace in Solar
-
Measuring
Semiconductor Device Input Parameters with Vector Analysis
- Computing with Scattering Parameters
- Measurements with Scattering
Parameters
- Ponderings on Power Measurements
- Scattered Thoughts on
Scattering Parameters
Sunshine Design Engineering Services
23517 Carmena Rd
Ramona, CA 92065
760-685-1126
Featuring: Test Automation Services, RF Calculator
and S-Parameter Library (DLL & LLB)
LinkedIn:
Joe Cahak
SunshineDesign@cox.net
Posted December 15, 2013









 to convert between Noise Power and Temperature. See figure3 for Te/Np curve.
to convert between Noise Power and Temperature. See figure3 for Te/Np curve. .
.
 .
.
 .
It has a decreasing energy per Hz as frequency increases.
.
It has a decreasing energy per Hz as frequency increases.  .
It has faster decreasing energy per Hz as frequency increases as pink noise. These first three noise colors are considered
power-law noise as they follow a power law noise energy/frequency distribution White having a 0 exponent, pink a 1
and Brown an exponent of 2.
.
It has faster decreasing energy per Hz as frequency increases as pink noise. These first three noise colors are considered
power-law noise as they follow a power law noise energy/frequency distribution White having a 0 exponent, pink a 1
and Brown an exponent of 2.

 =
=
 in SI base units with an uncertainty of Ur=0.71ppm about half of the last best measurement. See
in SI base units with an uncertainty of Ur=0.71ppm about half of the last best measurement. See







 .
The
.
The
 is the signal to noise ratio of the system calibration. The
is the signal to noise ratio of the system calibration. The
 is
the signal to noise ratio of the system and DUT total response. This is more typically used in the Log format Noise
Figure in NFdB which can be computed from the formula
is
the signal to noise ratio of the system and DUT total response. This is more typically used in the Log format Noise
Figure in NFdB which can be computed from the formula
 .
The inverse of this can be computed from
.
The inverse of this can be computed from
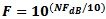


 or Irms =
or Irms =

 or
or  when
the temperature of the system under test is not at Te=290K.For a receiver with a S/N performance level the MDS formula
adds the S/N factor
when
the temperature of the system under test is not at Te=290K.For a receiver with a S/N performance level the MDS formula
adds the S/N factor
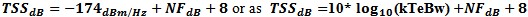
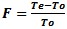 or
or
 or Te = To(F-1).
or Te = To(F-1). for when user is using noise figure in log units.
for when user is using noise figure in log units.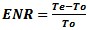 or
or 
 or
or  with
To=290K
with
To=290K off with Np on must be greater than Np off to compute a noise figure. Then the Y ratio can be used to compute the
Noise Figure in dB. Be sure to use sample detector mode when making these measurements. Video Averaging will reduce
the specular noise by averaging across frequency to smooth to the video average.
off with Np on must be greater than Np off to compute a noise figure. Then the Y ratio can be used to compute the
Noise Figure in dB. Be sure to use sample detector mode when making these measurements. Video Averaging will reduce
the specular noise by averaging across frequency to smooth to the video average. or
or 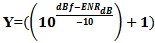
 from
which we derive
from
which we derive  .
.
 in
linear units of F=Noise Factor and G=Gain in linear units.
in
linear units of F=Noise Factor and G=Gain in linear units.
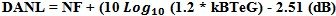



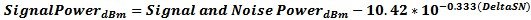

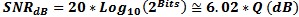 for
Arbitrary Waveforms
for
Arbitrary Waveforms for
Sine Waveforms
for
Sine Waveforms

 or
or  or
or 
 to convert to dBF. This can be used to compute the noise figure of a DUT embedded inside a test fixture. Keep in mind
T can be substituted in for F using Te=To(F-1) in the equation for cascaded noise calculations. We will see these
formulas again with input and output loss correction of DUT measurements. We will also see this in the cold power
noise measurement technique.
to convert to dBF. This can be used to compute the noise figure of a DUT embedded inside a test fixture. Keep in mind
T can be substituted in for F using Te=To(F-1) in the equation for cascaded noise calculations. We will see these
formulas again with input and output loss correction of DUT measurements. We will also see this in the cold power
noise measurement technique.

 from
which we compute the noise factor
from
which we compute the noise factor  .
.




 . The + and – represent the max and minimum mismatch for the measurement mismatch loss of power measured. ρg and
ρl are the generator and load reflection coefficient. This would be an input loss direct error factor
on the measurement that is F1 in the cascade noise equation along with the input network loss added for the total
loss.
. The + and – represent the max and minimum mismatch for the measurement mismatch loss of power measured. ρg and
ρl are the generator and load reflection coefficient. This would be an input loss direct error factor
on the measurement that is F1 in the cascade noise equation along with the input network loss added for the total
loss.


 T1=DUT, Lin = Loss, Tl = Loss effective temperature in K.
T1=DUT, Lin = Loss, Tl = Loss effective temperature in K.
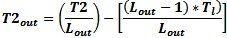
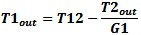

 n
n
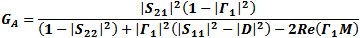















 with
ENB=Rcvr Noise BW, fb=bit rate
with
ENB=Rcvr Noise BW, fb=bit rate 
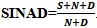 .
In practice the power is detected for the input signal for the S+N+D. For the N+D the signal is filtered with a tight
signal frequency filter and the Noise and Distortion components are left to use to divide the total power by for the
resulting SINAD. When measuring a receiver audio output the bandwidth of the SINAD measuring instrument can give varying
results depending on how wide the noise floor extends in frequency. If the audio output is integrated for SINAD over
a 3 kHz bandwidth vs. over a say 20 kHz bandwidth the SINAD will be much lower for the wideband noise case
verse the narrow band audio noise case. Most receiver audio channels are not very wide in bandwidth for signal
transmission efficiency. So, if having trouble with a receiver, measuring SINAD, check the measurement bandwidth.
In the case shown in figure 31 and 32, the impact of the wideband audio noise on a SINAD measurement is demonstrated.
In this case SINAD went from 16.4 dB in the 20 kHz noise case to 18.4 dB in the 3 kHz noise bandwidth
case. In both cases the S/N ratio was approximately equal. So the difference was just from the added noise bandwidth.
.
In practice the power is detected for the input signal for the S+N+D. For the N+D the signal is filtered with a tight
signal frequency filter and the Noise and Distortion components are left to use to divide the total power by for the
resulting SINAD. When measuring a receiver audio output the bandwidth of the SINAD measuring instrument can give varying
results depending on how wide the noise floor extends in frequency. If the audio output is integrated for SINAD over
a 3 kHz bandwidth vs. over a say 20 kHz bandwidth the SINAD will be much lower for the wideband noise case
verse the narrow band audio noise case. Most receiver audio channels are not very wide in bandwidth for signal
transmission efficiency. So, if having trouble with a receiver, measuring SINAD, check the measurement bandwidth.
In the case shown in figure 31 and 32, the impact of the wideband audio noise on a SINAD measurement is demonstrated.
In this case SINAD went from 16.4 dB in the 20 kHz noise case to 18.4 dB in the 3 kHz noise bandwidth
case. In both cases the S/N ratio was approximately equal. So the difference was just from the added noise bandwidth.